The simultaneous equations are also known as the system of equations, in which it consists of a finite set of equations for which the common solution is sought. To solve the equations, we need to find the values of the variables included in these equations. So simultaneous equations are those equations which are correct for the certain values of unknown variables at a same time. We can solve such a set of equations using different methods. Let us discuss different methods to solve simultaneous equations in the next section.
Cross-Multiplication Method for Solving Simultaneous Equations
Jon bought one packets of chips so, amount for chips will be ‘x’ and he bought seven packets of biscuits, amount for biscuits will be ‘7y’. Since values of x and y satisfy both equations, so our solution is correct. \(x\) and \(y\) values can be found which will solve both of the original equations at the same time or simultaneously. An equation with two unknown values will have infinitely many solutions. Subtracting the second equation from the first equation leads to a single variable equation. Use this equation to determine the value of y , then substitute this value into either equation to determine the value of x .
How do you solve pairs of simultaneous equations?
You can solve simultaneous equations by adding or subtracting the two equations in order to end up with an equation with only one unknown value. Linear simultaneous equations are usually solved by what’s called the elimination method (although the substitution method is also an option for you). You’ll learn what simultaneous equations are and how to solve them algebraically.

What are Simultaneous Equations?
We can find the value of x by dividing 2 on both sides, but sometimes problems give the two or more equations. These equations involve two or more unknown variables, as x is an unknown value in above equation, which we have to determine. Simultaneous linear equations are the system of two linear equations in two or three variables that are solved together to find a common solution. However when we have at least as many equations as variables we may be able to solve them using methods for solving simultaneous equations. The simultaneous equation is an equation that involves two or more quantities that are related using two or more equations.
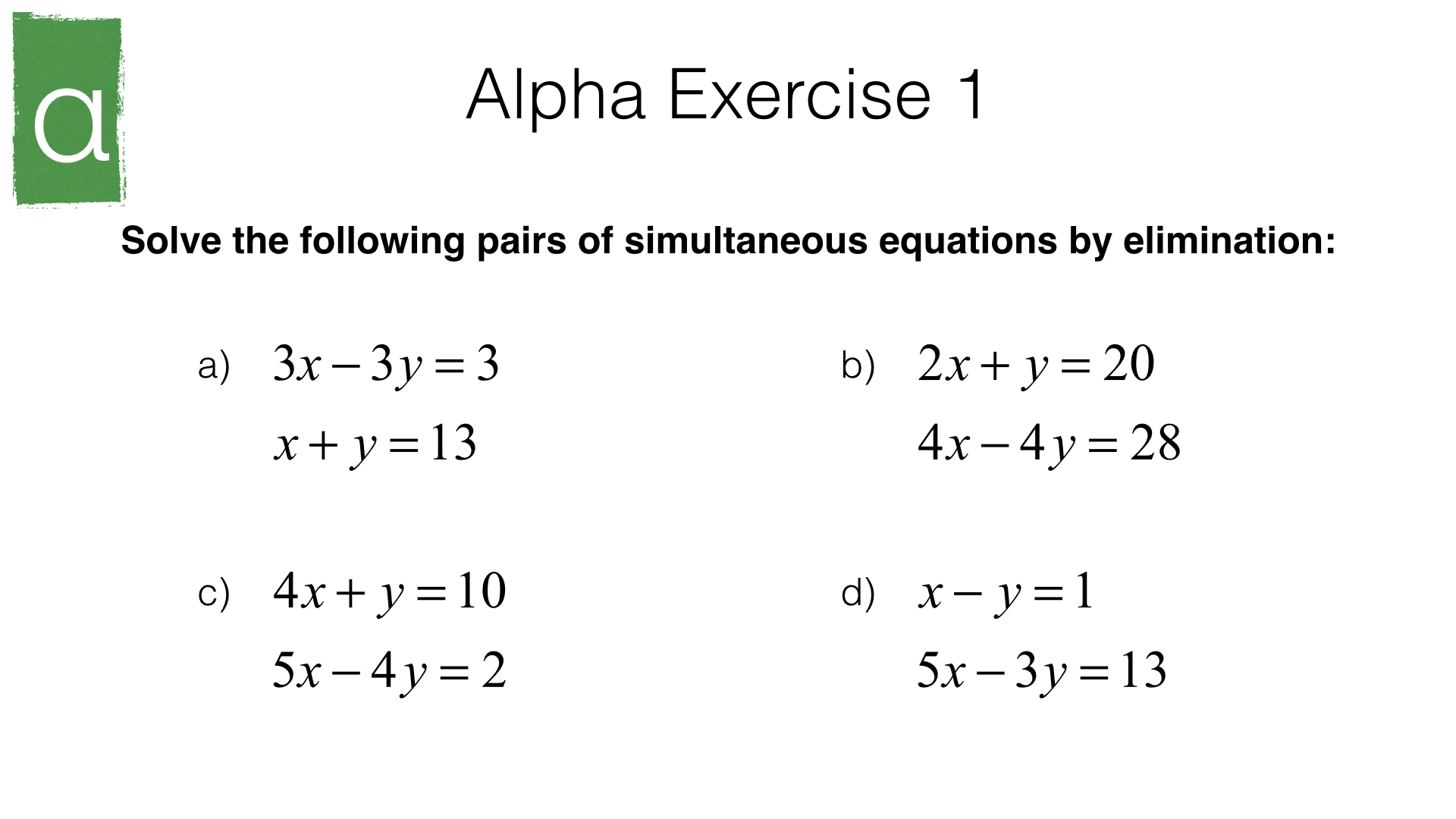
Elimination Method
We will also discuss their relationship to graphs and how they can be solved graphically. Nonlinear simultaneous equations are those equations in which power of at least one unknown variable must be greater than one. Simultaneous equations require algebraic skills to find the values of letters within two or more equations. They are called simultaneous equations because the equations are solved at the same time. In this article, we are going to discuss the simultaneous equations which involve two variables along with different methods to solve.
- The common type of equations in mathematics is linear equations, non-linear equations, polynomials, quadratic equations and so on.
- Neither the \(x\) nor the \(y\) will be eliminated by adding or subtracting these equations as they stand.
- To solve simultaneous equations, we need the same number of equations as the number of unknown variables involved.
- A system of two or more equations with two or more unknown variables solved at the same time is called simultaneous equations.
- Go through the following problems which use substitution and elimination methods to solve the simultaneous equations.
What are simultaneous equations?
Simultaneous equations can have no solution, an infinite number of solutions, or unique solutions depending upon the coefficients of the variables. We can also use the method of cross multiplication and determinant method to solve linear simultaneous equations in two variables. We can add/subtract the equations depending upon the sign of the coefficients of the variables to solve them. Let us now understand how to solve simultaneous equations through the above-mentioned methods.
Sometimes equations need to be altered, by multiplying throughout, before being able to eliminate one of the variables (letters). In this case, a good strategy is to multiply the second equation by 3 . We can then subtract the second equation from the first to leave an equation with a single variable. Once this value is determined, we can substitute it into either equation to find the value of the other variable.
A linear equation contains terms that are raised to a power that is no higher than one. Simultaneous equations are two or more algebraic equations that share variables such as x and y. Jon bought three packets of chips so, amount for chips will be ‘3x’ and he bought two packets of biscuits, amount for biscuits will be ‘2y’. Let ‘x’ is the price of one packets of chips and ‘y’ is the price of one packet of biscuit. In this graph point A representing the point of intersection.
We will get the value of a and b to find the solution for the same. Go through the following problems which use substitution and elimination methods to solve the simultaneous equations. To solve simultaneous equations, we need the same number of equations as the number of unknown variables involved. We shall discuss each of these methods in detail in the upcoming sections with examples to understand their applications properly. This is another approach to solving simultaneous linear equations in two variables.
The unknowns of \(x\) and \(y\) have the same value in both equations. This fact can be used to help solve the two simultaneous equations at the same time and find the values of \(x\) and \(y\). First of all, we draw the graph of both equation one by one and then trace out the intersection gambling winnings of lines, which will be the our required solution. Since it is a quadratic equation in term of ‘y’, we can solve it by factorization. In this step ‘x’ eliminates, we get the equation in term of ‘y’ only. You need to draw the graphs of the two equations and see where they cross.
Here, a1 and a2 are the coefficients of x, and b1 and b2 are the coefficients of y, and c1 and c2 are constant. The solution for the system of linear equations is the ordered pair (x, y), which satisfies the given equation. Have you ever had a simultaneous problem equation you needed to solve? When you use the elimination method, you can achieve a desired result in a very short time. This article can explain how to perform to achieve the solution for both variables. From equation (1) and equation (2) we will determine the value of x and y.
